|
| |
nextnano3 - Tutorial
3D Tutorial
Quantum dot molecule
Author:
Stefan Birner
==> This is the old website:
A new version of this tutorial can be found
here.
==> 3DQD_molecule_cuboid_asymmetric_nn3.in / *_nnp.in - input file for the nextnano3 and nextnano++
software
Quantum dot molecule
- In this tutorial, we study two coupled quantum dots (QDs), i.e. two
"artificial atoms" that form an "artificial molecule".
The two QDs are asymmetric and differ with respect to their height (4 nm and
6 nm).
- We keep things simple by using cuboidal shaped GaAs QDs surrounded by Al0.35Ga0.65As
barriers, i.e. we neglect strain and piezoelectric effects which is
reasonable as the two materials GaAs and Al0.35Ga0.65As
have pretty similar lattice constants.
We also neglect the wetting layers and excitonic effects.
- In order to keep the CPU time to a minimum, we do not use the k.p
approximation, i.e. we use for both electrons and the heavy hole a
single-band effective mass approximation for the Schrödinger equation
(parabolic and isotropic effective mass tensor).
Nevertheless, this is sufficient to show some basic quantum physical effects
of this QD molecule.
We use different electron and hole masses in the barrier and well material,
respectively.
- The left QD has the dimensions 10 nm x 10 nm x 4 nm
(smaller dot).
The right QD has the dimensions 10 nm x 10 nm x 6 nm (larger
dot).
The two QDs are separated by a 2 nm Al0.35Ga0.65As
barrier.
- The grid resolution is 0.5 nm (rectangular tensor grid). This leads to a
3D Schrödinger matrix of dimension 50,225.
- We apply Dirichlet boundary conditions to the Schrödinger equation, i.e.
the wave functions are allowed to penetrate the following distances into the
barrier material (on each side):
- along the x and y directions: 4 nm
- along the z direction: 4.5 nm
- We vary the electric field along the growth direction (z axis) in steps
of -2.5 kV/cm, i.e. from 0 kV/cm to -40 kV/cm.
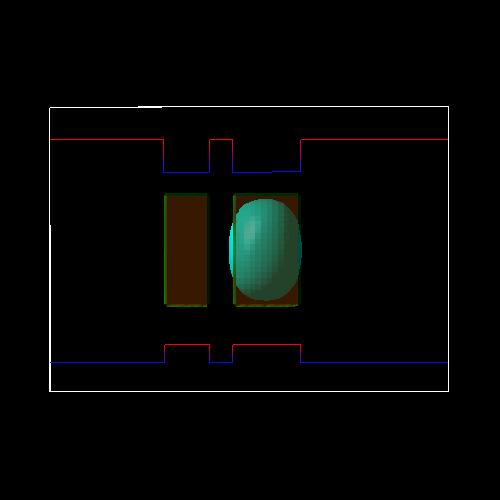
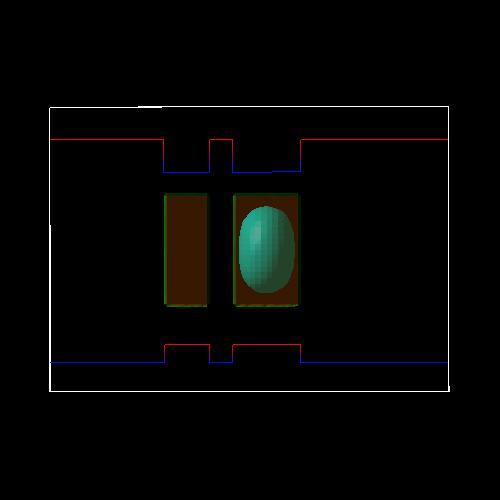
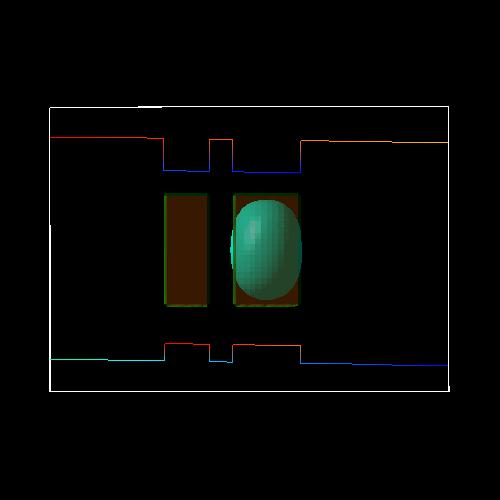
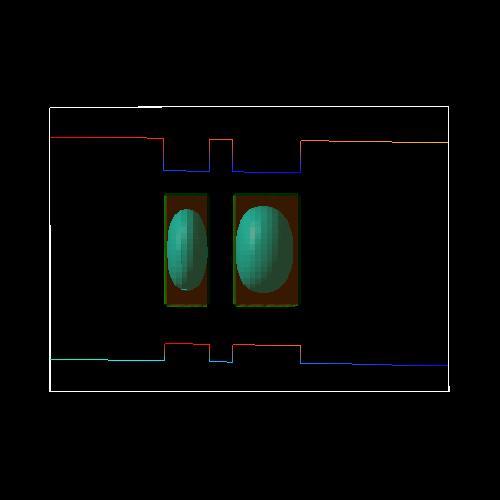
- The following figures show the square of the electron (left side) and
heavy hole (right side) wave functions (isovolumes of 25 % of psi2)
for different applied electric fields (0 kV/cm, -17.5 kV/cm, -40 kV/cm).
A slice through the conduction and valence band edges along the growth
direction through the center of the QDs is also shown.
|
 |
 |
|
electron ground state for zero electric
field |
heavy hole ground state for zero
electric field |
At zero applied
electric field, both electron and heavy hole are located in the larger dot
and
form a direct (bright) exciton. |
| |
|
| |
|
|
 |
 |
|
electron ground state for an electric
field of -17.5 kV/cm |
heavy hole ground state for an electric
field of -17.5 kV/cm |
|
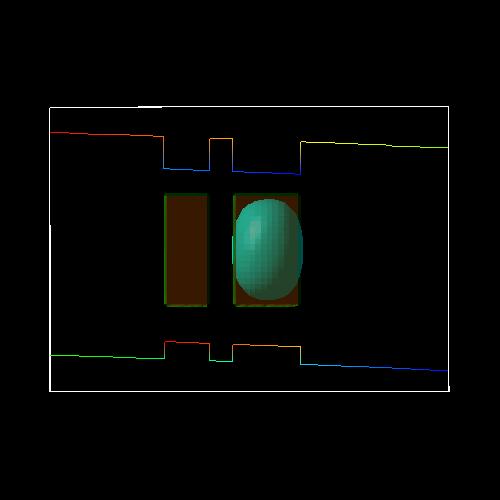
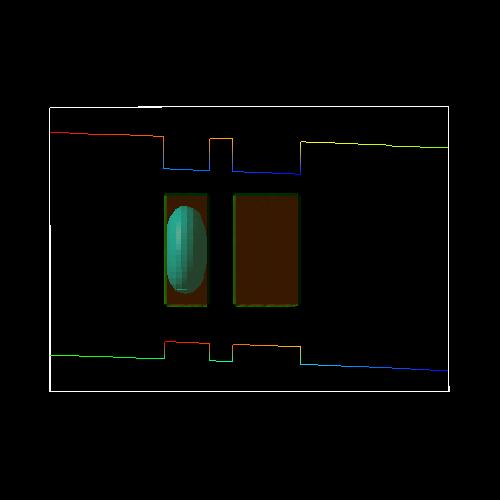
At an electric field of -17.5 kV/cm, the
electron is still located in the larger dot on the right side,
whereas the heavy hole forms a bonding (right figure) and an antibonding
state (not shown) and is thus located in both wells (strong coupling).
The exciton that is formed is something in between a direct and an indirect
exciton. |
| |
|
| |
|
|
 |
 |
|
electron ground state for an electric
field of -40 kV/cm |
heavy hole ground state for an electric
field of -40 kV/cm |
|
At an electric field of -40 kV/cm, the electron
is still located in the larger dot on the right side,
whereas the heavy hole ground state is now located in the left QD.
An indirect (dark) exciton is formed.
The exciton is called dark because the electron-hole overlap is much smaller
and
thus its oscillator strength (probability of optical transition) is much
weaker (see figure below on spatial electron-hole overlap integrals). |
| |
|
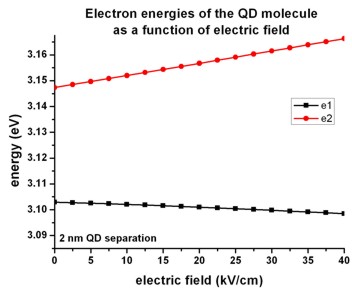
- The following figure shows the electron energies of the ground state (e1)
and the first excited electron state (e2)
of the QD molecule.
The ground state (e1) is always located in the larger QD (right side)
whereas the first excited electron state (e2)
is always located in the smaller QD (left side).
The third and the forth eigenstate (e3, e4) are degenerate (not shown)
because our QD molecule has a symmetry with respect to the x and y
coordinates. They are always located in the right QD.
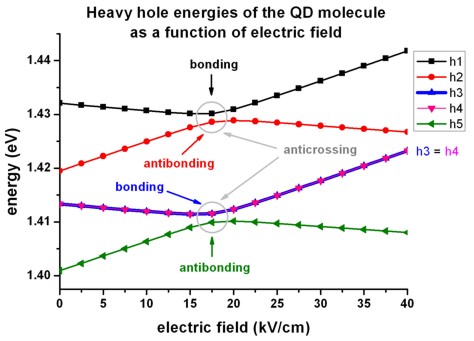
- The following figure shows the heavy hole energies of the ground state (h1)
and the excited hole states (h2,
h3,
h4,
h5) of the QD molecule.
In contrast to the electrons, the hole coupling between the two QDs is much
stronger due to the smaller barrier height.
At -17.5 kV/cm anticrossing between the states occur due to the formation of
bonding and antibonding states (see figures of the hole wave functions
further below).
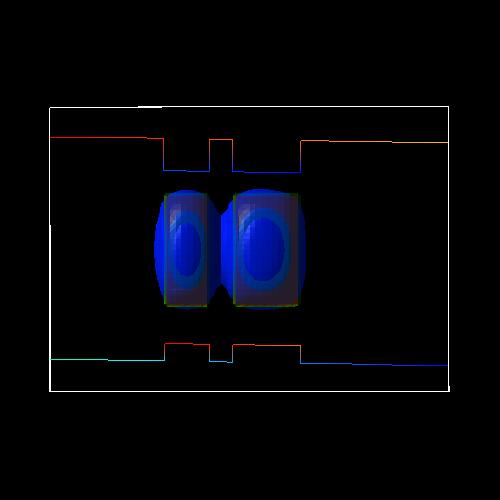
- The following figure shows the square of the lowest five hole
wave functions (isovolumes of 2 % of psi2) at an electric field of
-17.5 kV/cm.
|
 |
 |
|
heavy hole (h1) ground
state at -17.5 kV/cm (bonding)
|
heavy hole (h2)
first excited state at -17.5 kV/cm (antibonding)
|
|
 |
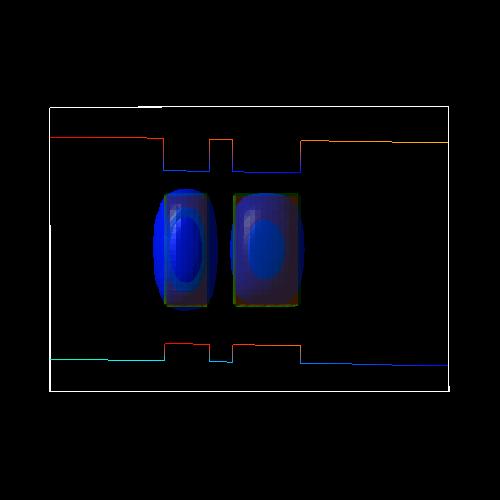
 |
|
heavy hole (h3)
state at -17.5 kV/cm (bonding) |
heavy hole (h4)
state at -17.5 kV/cm (bonding) |
|
Note that heavy hole (h3)
and heavy hole (h4) are
degenerate due to the symmetry with respect to the x and y axis.
|
|
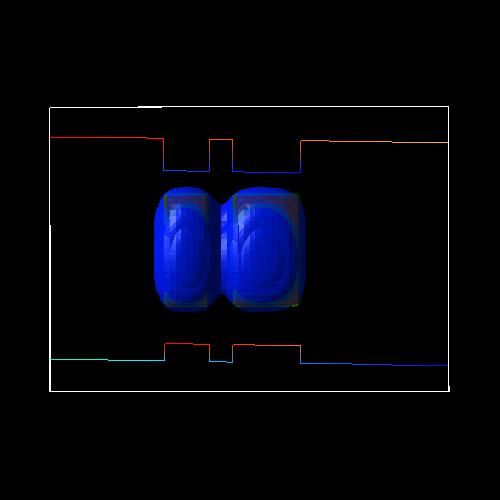
 |
|
|
heavy hole (h5)
state at -17.5 kV/cm (antibonding)
|
heavy hole (h6) state at
-17.5 kV/cm is also antibonding and
degenerate with (h6) due to (x,y) symmetry (not shown) |
| |
|
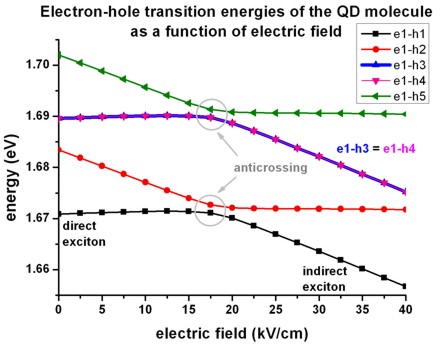
- The following figure shows the five lowest electron-hole transition
energies of the QD molecule as a function of electric field.
For fields smaller than -17.5 kV/cm a direct (bright) exciton is the ground
state (both electron and hole wave function are located in the larger QD
(right side), whereas for fields larger than -17.5 kV/cm an indirect (dark) exciton is the ground state where the electron is located in the larger QD
(right side) and the hole is located in the smaller QD (left side).
Therefore, the nature of the QD molecule ground state changes from direct
to indirect.
At -17.5 kV/cm anticrossings occur due to the bonding and antibonding
character of the hole wave functions.
- To understand the strength of the optical transitions we have to
evaluate the matrix elements of the envelope functions, i.e. the spatial
overlap integral over the electron and hole wave functions.
integral (psiel,i*(x) psihl,i(x) dx)
As expected, the direct exciton is much brighter than the
indirect (dark) exciton.

- Some of the material parameters that are used in this tutorial are based on the paper of
M. Grundmann, D. Bimberg
Formation of quantum dots in twofold cleaved edge
overgrowth
Phys. Rev. B 55 (7), 4054 (1997).
i.e. they are the same as in the 3D CEO QD
tutorial (apart from the effective masses).
|